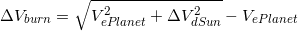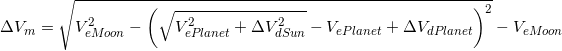When visiting an extraterrestrial moon, is it typically most efficient to fly straight into the moon's orbit then do a capture burn?

Question

Consider the mission of travelling from Kerbin to Ike (low orbit). There are two ways of getting to Ike that I'm trying to compare in terms of fuel requirements.

First method: Duna capture, Ike flyby

1. Leave Kerbin and get a Duna flyby.
2. Fine-tune Duna flyby for a low Duna periapsis.
3. Burn retrograde at the Duna periapsis to achieve an elliptical orbit.
4. Wait for a good window then fly by Ike, adjusting the current orbit if needed.
5. Fine-tune Ike orbit for a periapsis at low orbit.
6. Burn retrograde at periapsis to achieve low Ike orbit.

Second method: direct Ike flyby and capture

1. Leave Kerbin and get a Duna flyby.
2. Fine-tune Duna flyby for an Ike flyby + low Ike periapsis.
3. Burn retrograde at the Ike periapsis to achieve low Ike orbit.

Which scenario requires less ΔV? Is this typical for other Kerbin -> E.T. Moon trips? Are there any corner cases or exceptions to the norm (e.g. high/low planet:moon mass ratios, Laythe, high/low spacecraft eccentricity around Kerbol, etc)?

Answer 1

Third method is better

Oberth effect + Aerobraking + Gravity assist.

1. Aerobraking is free braking. Only one moon, Laythe, have atmosphere. Eve, Duna, Jool and Kerbin, which tend to be most interesting planets, can easily capture you with aerobraking. Free Delta-V.

Aerobraking happens at low PE so it will be coupled with Oberth effect. Aerobraking alone will save you enough Delta-V to encounter moon later on, with some planing you can even encounter it for free. Quick saving and Trajectories mod are advised. Of course it will require craft capable of aerobraking, inflatable heat shield is good starting point.

2. Oberth effect (as simulated in KSP) makes braking more efficient at high speed. Highest speed in orbit is at PE. More massive body means higher speed at PE. Which means more saving from Oberth effect. Therefore main body is better for braking.

You might want to have high thrust-to-weight ratio, long burn will waste propellant. But try not to hurt your total Delta-V. Before you go for low PE on body without atmosphere, check its highest point. You do not want to encounter mountain at 5000 m/s.

3. Gravity assist will change your orbit virtually for free. You can use moon encounter to change PE at main body encounter. Which will give you aerobraking and Oberth effect.

So in perfect encounter you:

1. Bounce on moon
2. Skim main body, brake or aerobrake
3. Encounter moon again
4. Expend laughable amount of delta-v to circularize moon orbit.

---

Capture burn from interplanetary transfer involves braking, a lot of it. Delta-V requirements tend to be in thousands. Savings in capture burn tend to be higher than moon encounter burns. Do not forget mid course corrections, long before planetary rendezvous you can make high changes in planetary orbit for very low delta-V cost, for example changing orbit from prograde to retrograde will be in tens of m/s instead of thousands.

Jool is an exception, as always.

Laythe and Tylo have 80% of Kerbin mass, which gives them notable gravity assist and Oberth effect. Those moons can capture you with nothing more than gravity assist and some luck in alignment. Orbit changes on Jool itself will cost a lot, due to its massive size, so use moons as much as you can.

---

Example of aggressive Ike/Duna transfer

I found some time to play so here is my take at red planet.

I went with lowest Delta-V Hohmann-style transfer so Duna PE at encounter was rather low ~1500 m/s.

With some orbit tweaking, and bucket of luck, I got direct encounter at Ike with low Duna PE. Quite good starting point for tests.

Have in mind that:

1. Ike low orbit <-> Duna low orbit switch is somewhere between 350m/s and 600m/s, depending on starting and target orbits, both ways (half for transfer, half for circular orbit).
2. Escaping Duna sphere of influence costs around 130m/s from Ike low orbit, while from Duna low orbit it is around 350m/s

Plan your mission accordingly if you have return in plans. If you want to visit both Duna and Ike, aerocapture on Duna would be cheapest in Delta-V overall. Especially when you consider that Duna escape craft can easily be capable of Ike landing.

(I simply can not give precise numbers because it heavily depends on your orbit and planetary alignment)

I used Duna PE to tweak Ike PE for ~100m/s. Ike low orbit needed ~900m/s to circularize.

Now that's some orbital billiard. ~700 m/s to capture in Duna low orbit, coupled with cheap orbital plane change by bouncing on Ike (300m/s, same change with engines alone would cost above 800m/s).

Ike gravity is too low for meaningful gravity assist, but here is one anyway:

Note: I had horrible position for Ike gravity assist, simply shooting through its sphere of influence, if ship trajectory would be tangent to Ike orbit it would be much more effective.

---

Afterword

In the end, it will mostly depend on your mission profile, especially your plans for return. You have lot of options, with plenty of possible combinations. Remember, even if you will use most effective transfers, you will waste fuel if orbits are not in line with your exploration plan. Plan in advance. And use Precise Maneuver Node Editor (MechJeb comes with one, but there are more to pick from).

If you want some fun ideas for Duna/Ike check out Duna Ore Bust web comic.

See you at Barsoom!

Answer 2

I ended up doing my own homework since I needed something very precise to use in my KSP mission planning calculator (the next update - v9 - will feature a sophisticated mission builder). Here are my findings.

It Depends

There are many factors at play here. The answer differs from moon to moon, as well as where the planet and moon are in their respective orbits, the properties of the spacecraft, and other factors.

The main concept that we're exploiting is that a burn during a hyperbolic orbit is amplified with respect to the primary body. This is illustrated by the following equation:

V² = V_esc² + V_∞²

Holding V_esc constant, a small change in V will result in a larger change in V_∞ because V is much larger than V_∞. Note that this is more pronounced for very high values of V_esc since V > V_esc for hyperbolic flight.

This needs to be used to "circularize" the orbit around a primary before stable elliptical orbit can be achieved around the satellite. During a moon flyby, this needs to be done twice: once for the sun-planet system, and once for the planet-moon system. And for a direct moon approach, the moon capture burn is used to accomplish both "circularizations".

Direct Moon Approach

If the moon has an atmosphere, great! then aerocapture, because ΔV will be pretty small. But, that's only the case for one moon in the game. For other moons, we can calculate what the burn will look like.

Consider the escape velocity at the periapsis of a planet ΔVePlanet, and let's say you had an elliptical orbit around the sun before entering the planet, and the cost (during interplanetary flight) of circularizing the solar orbit so that the spacecraft is going about the same speed as the planet (required for an elliptical flyby) is ΔVdSun. The cost of achieving ΔVdSun during the flyby at periapsis is given by:

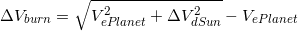
Brief explanation: V² = V_esc² + V_∞², but during a hyperbolic flight at periapsis, V = V_esc + V_extra. Therefore V_extra = (V_esc² + V_∞²)^0.5 - V_esc. We want to kill the extra V so we can switch to a sub-parabolic trajectory.

This ΔVburn can be effected by a moon burn, but there is an additional amount of ΔV required: the amount to "circularize" the planetary orbit to match the moon's, ΔVdPlanet

Thus far, I've been saying the word "circularize" in quotes because some planets and moons are elliptical. This greatly complicates things because it means that even at the height of low stable orbit, the burns require to go from hyperbolic orbit to parabolic orbit can vary widely.

It's much more efficient to do these capture burns at the periapsis of moons' orbits around their planets, especially when the planet is at it's periapsis around the sun. Let's ignore that for now and assume the capture burns are constant (i.e. the average case) because in this game we don't have advanced tools or facilities or patience to account for this source of error.

The final burn amount is given by:

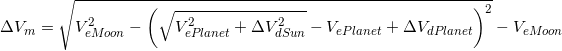
This is not the burn required to get into low stable orbit, it's the burn required to get into a parabolic moon orbit. This can be compared with the result from below.

Planet Capture, Moon Flyby, Moon Capture

Basically we can use the first equation to obtain a sub-parabolic orbit around the planet (or a smaller amount, for planets with atmospheres if we're willing to aerocapture), then add a little bit of ΔV to adjust the orbit for a moon flyby, and finally add the amount of ΔV required to switch to an elliptical orbit on that moon.

Note that the hardest term here to calculate is ΔVdPlanet because it depends on where the moon is in it's orbit, as well as other orbital properties.

Conclusion

The choice of whether or not to do a direct moon flyby/capture essentially depends on the comparison between the above two equations. There are values that could be used that would make either option better depending on the situation, for instance, a very fast-moving spacecraft (relative to the target planet) travelling to a small moon on a large planet might have better luck doing the planet capture first. However a slow-moving spacecraft travelling to a very massive moon can probably get away with a direct moon encounter + capture.

There are also some unknown/unknowable variables such as:

• The ΔV required to go from an elliptical orbit around a planet to a low-periapsis moon flyby (since it by itself depends on many other factors including player patience, avoiding collisions with other moons, the moon's orbital position upon intercept, etc).
• The ISP of the engine being used (calculations assume an instantaneous change in velocity at periapses, hence long burns waste fuel).
• The orbital position of both the planet and moon upon arrival.
• etc.

Also there are various risk factors to consider, especially regarding aerocaptures.

I won't go farther than that because an in-depth analysis is outside of the scope of this question/site and not in the spirit of video games.